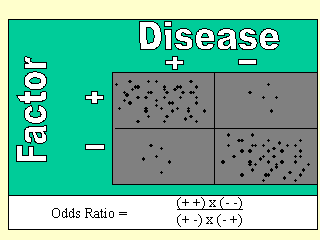
In the diagram, each dot represents an individual. Each appears in only one of the boxes either + for both disease and factor, or – for both disease and factor, or + - or - +. There are clearly many individuals in the ++ and - - boxes, but few in the others. This demonstrates a very strong relationship between the disease and the factor, both either being present or absent in most of the individuals. Statisticians demonstrate the probability that the relationship is serious by multiplying the + + box and the - - box and dividing this total by the +- and -+ boxes multiplied together. This is called the Odds Ratio and any departure from one, either higher or lower, may indicate the association. A very large number, as in this case, would indicate that the probability of this finding being a fluke or mistake would be very small indeed.

Odds Ratio =[(+ +) x (- -)]/[(+ -) x (- +)]